���˿���

One of the earliest and most important skills we all learn in maths is recognising and understanding place value; how much each digit is worth depending on where it is placed in a number. So, for example, the number 3333 is made up of:

3 thousands, 3 hundreds, 3 tens and 3 units

or

3000, 300, 30 and 3

Even though the digit (3) is repeated, it has a different value each time depending on what column it is in. This understanding of place value is at the heart of everything we do with numbers. Without it, the most basic sums would be impossible to carry out.

Place value is not only important for whole numbers; it extends to decimals too. A decimal point is used to separate whole numbers from fractions of numbers.

The columns on either side of the decimal point are infinite, but we usually work with units, tens, hundreds, thousands, tens of thousands and millions for whole numbers. For decimals, we focus on the columns tenths, hundredths and thousandths. By combing the two, ‘in-between’ numbers can be shown.

For example, we can say that the number 6.7 (‘six point seven’) is in-between 6 and 7; more than 6 but less than 7. We could go even further with the number 6.752. We can say that this number is accurate to three decimal places and is made up of 6 units, 7 tenths, 5 hundredths and 2 thousandths – the further down we go, the less value the digit has.

Zero hero!

It might come as a bit of a surprise, but one of the most important digits that we use every day is ‘0’. It is not worth anything, but it still has a very important job to do. Zero is used as a place holder – it keeps a space open in a column and lets us know that although there is nothing there at the moment, the column still exists. Without the zero in the number 5780, all of the other digits would have the wrong value as the number would become 578. We use the zero in the units column to keep all of the other digits in the correct place.

The importance of the zero can be seen when multiplying or dividing numbers by 10, 100 or 1000. Let’s take the number 624 as an example and multiply it by ten:

624 x 10 = 6240

You can see that the digits have not changed except for the extra zero. However, the value of the digits has increased. But how, and where did the zero come from? Well, when we multiply by ten, we are actually moving all of the digits one step to the left from the decimal point, which always stays in the same spot, like an anchor. This increases their value by one column. The zero that has appeared in the answer has actually moved from the right hand side of the decimal point.

The number 624 could actually be written as 0624.0, with a never ending set of zeros on either side of it. As these columns are not currently being used, we do not need to show these zeros; they might be invisible but they still exist, ready to come alive when needed. So, by multiplying 624 by 10, we have moved every digit one step to the left, creating a new space for the zero from the tenths column in the units column. The opposite of this happens when we divide the number by 10, with every digit moving one column to the right and decreasing in value:

624 ÷ 10 = 62.4

To multiply or divide a number by 100, we move every digit by two columns. For 1000, we move them by three columns. An easy way to remember this is by counting the zeros in the number – ten has one 0 so move one step; hundred has two 0’s so move two steps, one thousand has three 0’s so move…you guessed it, three steps.

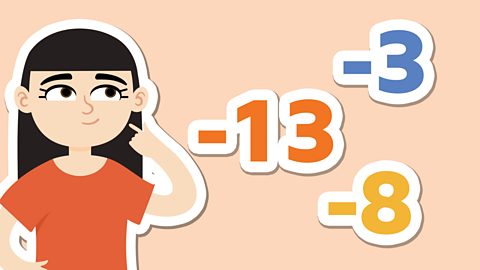
Try these examples below: