A geometric sequence goes from one term to the next by always multiplying or dividing by the same value.
The number multiplied (or divided) at each stage of a geometric sequence is called the common ratio.
Examples of geometric sequences are the frequencies of musical notes and interest paid by a bank.
Find out how notes on a keyboard relate to geometric sequences.
What is a geometric sequence?
Number sequences are sets of numbers that follow a pattern or a rule. If the rule is to multiply or divide by a specific number each time, it is called a geometric sequence.
The sound of a geometric sequence
Geometric sequences are important in music. Musical notes each have a frequency measured in Hertz (Hz). The higher the note, the higher the number of Hertz.
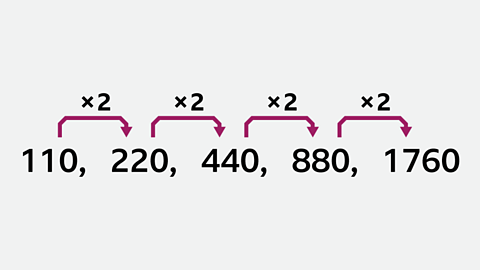
For example, the note A can be played with a frequency of 110 Hz, 220 Hz, 440 Hz, 880 Hz…
Each term has been multiplied by the same number to make the next term in the sequence.
Each of the specific frequencies is called a pitch. The interval between the pitches is called an octave.
The common ratio
The common ratio is the number you multiply or divide by at each stage of the sequence.
You can find it by dividing two consecutive pairs of terms. It doesn't matter which pair is chosen as long as they are next to each other:
220 Г· 110 = 2
440 Г· 220 = 2
880 Г· 440 = 2
The common ratio is therefore 2. You can find out the next term in the sequence by multiplying the last term by 2.

More on Algebra
Find out more by working through a topic
- count1 of 29
- count2 of 29
- count3 of 29

- count4 of 29